Dimensional Formula of Torque

The dimensional formula of Torque is 

[ M1  L2  T-2 ]

where [M], [L], and [T] are the fundamental quantities: Mass, Length, and Time.

From the above formula, we can derive the dimensions of torque as (1, 2, -1).

How to calculate the dimensional formula of torque?

We can derive this formula using the definition of torque.

In physics, we can define torque as the tendency of a force to rotate a body about some axis. So, mathematically, torque is given as:


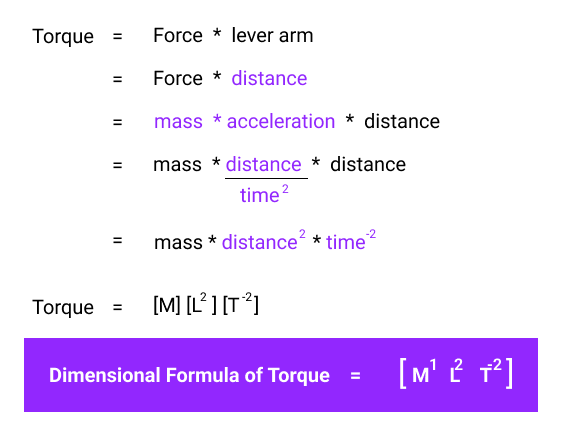
  Torque = Force * lever arm

Here, lever arm is the perpendicular distance from the axis of rotation to the line drawn along the direction of the force applied. Hence, we can also say


  Torque = Force * distance

We know that Force = mass * acceleration. Substituting the value of Force in the above formula, we get


  Torque = mass * acceleration * distance

We know 

  • acceleration = speed / time
  • speed = distance / time
  • acceleration = distance / time2

Therefore,


  Torque = ( mass * distance / time2 ) * distance

  Torque = ( mass * distance2 ) / time2

  Torque = mass1 * distance2 * time-2

Dimensionally, we use

  • [M] for mass
  • [L] for distance and displacement
  • [T] for time

Now the formula becomes


  Torque = [M1] * [L2] * [T-2]

Hence, the dimensional equation of torque is τ = [ M1 L2 T-2 ].

Derivation of the dimensional formula of torque using the formula.

Related Articles